Print preview

Temperature dependent viscosity and conductivity Problem Setup

A temperature dependent viscosity and conductivity model with slip and noslip boundary conditions was tested using the Sutherland model for perfect (constant specific heat) nitrogen. Again, as in the constant viscosity and conductivity model, the Clawpack and WENO methods were compared. The main difference of this test is the significant increase of the diffusive effects behind the Mach stem and around the shear/mixing layer. The viscosity and conductivity increase close to linearly with temperature, and the highest temperatures are between the Mach stem and the shear/mixing layer (aka the slipline in inviscid theory).

SutherlandModel

New Parameters for Nitrogen:

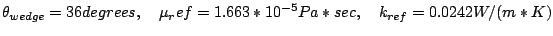
$\theta_{wedge} = 36 degrees, \quad \mu_ref=1.663*10^{-5} Pa*sec, \quad k_{ref} = 0.0242 W/(m*K)$

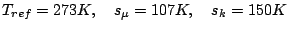
$T_{ref} = 273 K, \quad s_{\mu} = 107 K, }\quad s_{k} = 150 K$

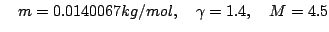
$\quad m = 0.0140067 kg/mol,\quad \gamma = 1.4, \quad M=4.5$

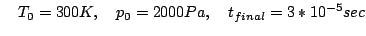
$\quad T_0=300 K, \quad p_0 = 2000 Pa, \quad t_{final}=3*10^{-5} sec$

24 Processors, SHC

For the following tests we used the computational domain: x = [-0.01,0.1], y = [0, 0.025]
Clawpack: base level 220x50 cells with levels refined 2,2,4,4 times respectively, max cfl = 0.9
WENO-TCD: base level 220x50 cells with levels refined 2,2,2,4 times respectively, max cfl = 0.85

64 Processors, SHC

For the following tests we used the computational domain: x = [-0.01,0.1], y = [0, 0.025]
5 levels: base level 110x25 cells with 4 more levels refined 2,2,4,4 times respectively

DoubleMachReflectionStudy

-- JackZiegler^? - 11 Aug 2008

End of topic
Skip to action links | Back to top

You are here: Amroc > DoubleMachReflectionStudy > VariablePropRotatedDMR

to top