Numerical Simulations of Gaseous Detonations

Multi-component Euler Equations

Reaction Terms of Detailed Chemistry

The reaction terms for detailed chemcial reaction are determined by a reaction mechanism of M chemcial reactions, i.e.

%MATHMODE{ \sum_{i=1}^K \nu_{ji}^f S_i {(A_j , \beta_j,E_j)\atop \rightleftharpoons} \sum_{i=1}^K \nu_{ji}^r S_i \qquad \qquad j=1,\dots,M\;. }%

The entire production rate for each species is then given by

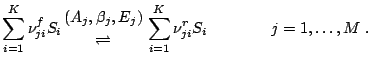
%MATHMODE{\dot \omega_i = \sum_{j=1}^M (\nu_{ji}^r-\nu_{ji}^f)\biggl[ k_j^f\prod_{n=1}^K\Bigl({\rho_n \over W_n}\Bigr)^{\nu_{jn}^f} - k_j^r\prod_{n=1}^K\Bigl({\rho_n\over W_n} \Bigr)^{\nu_{jn}^r} \bigg] \quad i=1,\dots,K\;, }%

where the reaction rates are calculated by the Arrhenius laws

%MATHMODE{ k_j^{f/r}(T) = A_j^{f/r} T^{\beta_j^{f/r}}\exp (-E_j^{f/r}/{\cal R}T)\;.}%

---

-- RalfDeiterding - 14 Dec 2004